Full H-vector Formulation

The FD-VBPM based on the E and H fields are equivalent and yield almost identical results [11].
Similar to the vector wave equation for the electric field, the equation for the magnetic field considering transversely scaled version of PML is:

here  .

The double-curl Equation 1 involves three vector components of the magnetic field, while strictly only two are needed.

Incorporating the divergence-free condition  into Equation 1, we can reduce the number of components in the field equation to the two transverse components of the magnetic field h_x and h_y only. To achieve this purpose, we separate the transverse and longitudinal components of Equation 1.

On the transverse plane, Equation 1 becomes:

Now, using an appropriate reference refractive index n₀ , and slowly varying envelope
approximation (SVEA), we assume the following form of the solution:

By using Equation 3 into Equation 2, we can recast Equation 1 in the following form:

with

From divergence condition, we get:

By using Equation 3 into Equation 6, we get:

By substituting Equation 7 into Equation 4, we get the following vectorial wave equation written in terms of the transverse components of magnetic field:

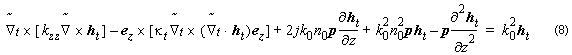
or

To obtain Equation 9, we assume that the permittivity along the propagation direction
is often slow, as is observed in real devices. Therefore, ∂ p ⁄ ∂z has been neglected.

Similar to the E-formulation, the elimination of the axial component using the
divergence condition.

 guarantees the complete elimination of spurious modes, and drastically reduced computation efforts and resources, compared to the formulation which uses three field components.

We can rewrite Equation 9:

where the operator Mh_t  is defined as:

Here, the matrix M can also be written in components:

The differential operators are defined by the following equations:

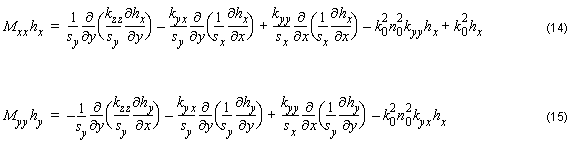
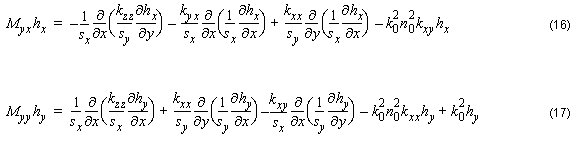
The discontinuities of ∂h_y ⁄ ∂x and ∂h_x ⁄ ∂y across the index interfaces along y and x directions are responsible for the polarization dependence ( i. e. h_xx ≠ h_yy ) and coupling ( i. e. h_xy  and h_yx ≠ 0 ).

The solution to Equation 9 can be written in a exponential form

which can also be approximated by a weighed finite-difference form

Here we get D = p

for paraxial approximation and

for wide angle-Pade(1,1).

As in the E-Formulation description, we can apply the Padé recursion formula

AS in E-formulation, we can derive wide-angle BPM considering high order Padé, as the parameter a is introduced to control the schemes used to solve the finite- difference equations.

We can recast Equation 19 in the following form

where h^l_t  and h_t ^{l + 1}  are field vectors at two sequential steps l and l + 1 , A and B are nonsymmetric complex band matrices. By solving Equation 23, we can simulate the propagation of the beam in anisotropic materials, such as the polarization dependence and coupling, due to both the material and geometrical effects.

The system in Equation 23 is solved efficiently by the well established sparse matrix solver “bicgstab” (BiConjugate Gradients Stabilized method ).