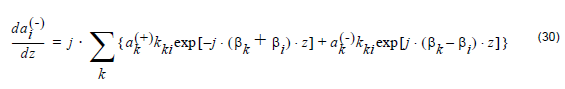Assume the electric field is a linear combination of the ideal modes (with no grating
perturbation), such that
where ai(+) and ai(-) are the slowly varying amplitudes of ith mode traveling in the +z
and –z directions. βi and ![]() is the propagation constant and modal field of the ith
is the propagation constant and modal field of the ith
mode.
The above electric field is used as trial solution in the Maxwell’s equation. The
following Coupled mode Equations (CMEs) can be derived by using the properties of
waveguide modes,
The coupling coefficient between modes k and i is given by:
where ![]() is the periodic refractive index perturbation of the grating,
is the periodic refractive index perturbation of the grating,
and n0(x, y) is the index profile of waveguide. n(x, y, z) is the grating index profile.
In OptiGrating, the coupled mode equations are based on non-orthogonal coupled
mode theory. Both the waveguide nature coupling and grating coupling are
considered. In order to formulate the coupled mode equations, waveguide modal
constants, fields, and coupling coefficients are calculated based on waveguide and
grating profiles. The coupled mode equations are then solved by the two mode or by
the multi-mode coupling formulation.